Page 92 - 《应用声学》2023年第2期
P. 92
280 2023 年 3 月
所有能量被镜像反射到一个方向,散射系数的数值 够实现所需要声散射体的 0 单元和 1 单元,并利用
为0。本文中用1 的角度间隔方式选取声压级数值, 其构建了声散射体。仿真计算结果表明,该声散射
◦
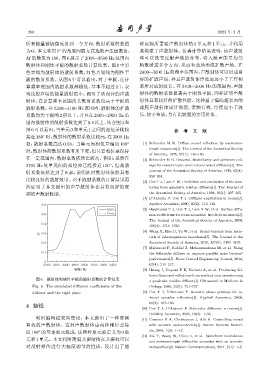
M 的数值为180。图6展示了2000∼3500 Hz范围内 体可以改变反射声场的分布,将入射声能量均匀
散射体和刚性平板的散射系数计算结果。图 6 中黑 地散射进多个方向,从而有效地形成扩散声场。在
色实线为散射体的散射系数,红色点划线为刚性平 2400∼3000 Hz的频率范围内,声散射体可以形成良
板的散射系数。从图 6 中可以看出,对于平板,在计 好的扩散声场,并且声散射体厚度远远小于工作频
算频率范围内散射系数都极低,基本不超过 0.1,表 率所对应的波长。在2400∼3000 Hz的范围内,声散
明反射声场的能量散射很小。而对于所设计的声散 射体的散射系数显著高于刚性平板,同样证明声散
射体,在计算频率范围内其散射系数均高于平板的 射体具有较好的扩散性能。这种基于编码超表面的
散射系数。在2300∼3100 Hz范围内,散射体的扩散 薄膜声散射体设计简便,宽频有效,厚度远小于波
系数均为平板的2 倍以上,并且在 2500∼2900 Hz范 长,便于布放,具有比较强的实用价值。
围内散射体的散射系数达到了0.4以上。结合图3和
图6可以看出,当单元 0和单元1之间的相位差比较 参 考 文 献
接近180 时,散射体的散射系数比较高,在3000 Hz
◦
处,散射系数高达 0.58。当单元间相位差偏离 180 ◦ [1] Schroeder M R. Diffuse sound reflection by maximum-
length sequences[J]. The Journal of the Acoustical Society
时,散射体的散射系数有所下降,但只要相位差保持
of America, 1975, 57(1): 149–150.
在一定范围内,散射系数依然比较高。例如,虽然在 [2] Schroeder M R. Binaural dissimilarity and optimum ceil-
2700 Hz 处单元间的相位差已经接近 120 ,但是散 ings for concert halls: more lateral sound diffusion[J]. The
◦
射系数依然达到了 0.46,说明此时散射体依然具有 Journal of the Acoustical Society of America, 1979, 65(4):
958–963.
比较良好的散射效果。对于散射系数的计算结果再 [3] Cox T J, Lam Y W. Prediction and evaluation of the scat-
次证明了本文提出的声学散射体在具有良好的宽 tering from quadratic residue diffusers[J]. The Journal of
频的声散射性能。 the Acoustical Society of America, 1994, 95(1): 297–305.
[4] D’Antonio P, Cox T J. Diffusor application in rooms[J].
0.6 Applied Acoustics, 2000, 60(2): 113–142.
MSD [5] Hargreaves T J, Cox T J, Lam Y W, et al. Surface diffu-
0.5 Plate sion coefficients for room acoustics: free-field measures[J].
The Journal of the Acoustical Society of America, 2000,
0.4
108(4): 1710–1720.
[6] Wang X, Mao D, Yu W, et al. Sound barriers from mate-
D 0.3
rials of inhomogeneous impedance[J]. The Journal of the
0.2 Acoustical Society of America, 2015, 137(6): 3190–3197.
[7] Mahmoud F, Kablief Z, Mohammadreza M, et al. Using
0.1 the Schroeder diffuser to improve parallel noise barriers’
performance[J]. Noise Control Engineering Journal, 2014,
0
2000 2200 2400 2600 2800 3000 3200 3400 62(4): 210–217.
ᮠဋ/Hz [8] Huang J, Dupont P E, Undurti A, et al. Producing dif-
fuse ultrasound reflections from medical instruments using
图 6 散射体和刚性平板的散射系数的计算结果 a quadratic residue diffuser[J]. Ultrasound in Medicine &
Fig. 6 The simulated diffusion coefficients of the Biology, 2006, 32(5): 721–727.
diffuser and the rigid plate [9] Cox T J, D’Antonio P. Acoustic phase gratings for re-
duced specular reflection[J]. Applied Acoustics, 2000,
60(2): 167–186.
4 结论
[10] Cox T J, D’Antonio P. Schroeder diffusers: a review[J].
Building Acoustics, 2003, 10(1): 1–32.
利用编码超表面理论,本文提出了一种宽频 [11] Cummer S A, Christensen J, Alù A. Controlling sound
有效的声散射体。这种声散射体由两种相位差接 with acoustic metamaterials[J]. Nature Reviews Materi-
近 180 的基本单元组成,这两种单元被定义为 0 单 als, 2016, 1(3): 1–13.
◦
[12] Xie Y, Wang W, Chen H, et al. Wavefront modulation
元和 1 单元。本文利用薄膜共振结构在共振处可以
and subwavelength diffractive acoustics with an acoustic
对反射相位进行大幅度调节的性质,设计出了能 metasurface[J]. Nature Communications, 2014, 5(1): 1–5.