Page 171 - 《应用声学》2023年第3期
P. 171
第 42 卷 第 3 期 宋乐等: 相位失配弹性平板复合波导中的缺陷态 609
0.470 进行实验验证。利用光学干涉方法高精度检测弹性
Mode1
Mode2 波模式 [19] ,将成为有效手段。总之,本文提出的弹
0.465
性波缺陷态调控方法不仅有利于波导的带隙分析
0.460
f/MHz 以及弹性波应力、应变场调控研究,也为声学滤波、
0.455
缺陷态特征模式分析、结构振动噪声衰减等声传播
0.450 控制领域提供重要参考和解决方案。
0.445
0 0.2 0.4 0.6 0.8 1.0
Dφ
参 考 文 献
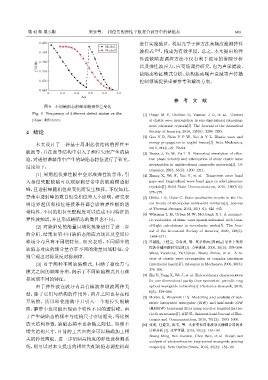
图 9 不同缺陷态的频率随相位差变化
Fig. 9 Frequency of different defect states vs the [1] Ponge M F, Croënne C, Vasseur J O, et al. Control
phase difference of elastic wave propagation in one-dimensional piezomag-
netic phononic crystals[J]. The Journal of the Acoustical
4 结论 Society of America, 2016, 139(6): 3288–3295.
[2] Guo Y B, Shim V P W, Yeo A Y L. Elastic wave and
本文设计了一种基于周期起伏结构的弹性平 energy propagation in angled beams[J]. Acta Mechanica,
2010, 214(1–2): 79–94.
板波导,并在波导结构中引入了相位失配产生的缺 [3] Zhang J, Ye W, Yu T X. Numerical simulation of effec-
陷,对透射谱禁带中产生的缺陷态特征进行了研究。 tive phase velocity and attenuation of shear elastic wave
结论如下: propagation in unidirectional composite materials[J]. Ul-
trasonics, 2013, 53(6): 1200–1211.
(1) 周期起伏弹性板中会形成弹性波禁带,引 [4] Zhang X, Wu F, Yao Y, et al. Transverse wave band
入相位失配缺陷可以观察到禁带中的缺陷模透射 gaps and longitudinal wave band gaps in solid phononic
crystals[J]. Solid State Communications, 2010, 150(5–6):
峰,且透射峰随相位差变化而发生频移。不仅如此,
275–279.
禁带中透射峰的数目也受相位差大小影响。研究表 [5] Ghiba I D, Gales C. Some qualitative results in the lin-
明边界起伏和相位连接条件都会影响弹性板的谱 ear theory of micropolar solid–solid mixtures[J]. Journal
of Thermal Stresses, 2013, 36(4–6): 426–445.
带特性,不同的相位失配程度可以造成不同特征的
[6] Wiseman L M, Urban M W, McGough R J. A paramet-
弹性波频谱,并且形成缺陷态的数目也不同。 ric evaluation of shear wave speeds estimated with time-
(2) 对缺陷处的能量局域化现象进行了进一步 of-flight calculations in viscoelastic media[J]. The Jour-
nal of the Acoustical Society of America, 2020, 148(3):
的分析,结果表明不同缺陷态的应力场以及空间位
1349–1371.
移场分布具有不同的特征。研究表明,不同频率的 [7] 汪越胜, 于桂兰, 章梓茂, 等. 复杂界面 (界面层) 条件下的弹
缺陷态对应的场分量存在不同的能量局域特征,分 性波传播问题研究综述 [J]. 力学进展, 2000, 30(3): 378–390.
Wang Yuesheng, Yu Guilan, Zhang Zimao, et al. A re-
别呈现出对称及反对称规律。 view of elastic wave propagation at complex interfaces
(3) 对于两种不同缺陷模式,归纳了相位差与 (interfacial layers)[J]. Advances in Mechanics, 2000, 30(3):
模式之间的频率分布,揭示了不同缺陷模式具有频 378–390.
[8] Zhi Y, Yang X, Wu J, et al. Extraordinary characteristics
移周期不同的特征。 for one-dimensional parity-time-symmetric periodic ring
由于弹性波在波导内具有横波和纵波两种类 optical waveguide networks[J]. Photonics Research, 2018,
6(6): 579–586.
型,除了它们与结构的作用外,两者之间也存在相
[9] Moitra S, Bhowmik P S. Modelling and analysis of sub-
互转换。所以即使结构中只引入一个相位失配缺 strate integrated waveguide (SIW) and half-mode SIW
陷,禁带中也可能出现两个特性不同的透射峰。由 (HMSIW) band-pass filter using reactive longitudinal pe-
riodic structures[J]. AEUE - International Journal of Elec-
于产生缺陷态的频率与结构尺寸密切相关,等比例
tronics and Communications, 2016, 70(12): 1593–1600.
放大结构参数,缺陷态频率也会随之降低。即便不 [10] 张明, 任建文, 陈文, 等. 光折变长周期波导光栅耦合器的设
增大结构尺寸,目前的工艺也完全可以精确加工相 计和分析 [J]. 光学学报, 2015, 35(3): 153–161.
Zhang Ming, Ren Jianwen, Chen Wen, et al. Design and
关的弹性薄板。进一步研制高精度的弹性波检测系
analysis of photorefractive long-period waveguide grating
统,则可以对本文提出的相位失配缺陷态调控机理 coupler[J]. Acta Optica Sinica, 2015, 35(3): 153–161.